
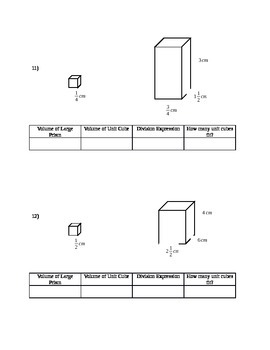
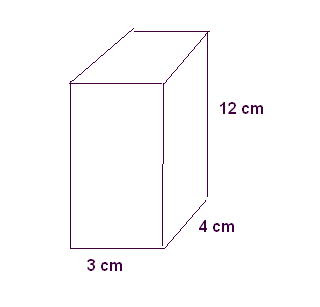
What would the volume of this triangular prism be? If students are having difficulty prompt them with: What would the volume of this triangular prism be if it is created by halving the rectangular prism? (The volume will be 8 cm 3.) Now ask students what shape they would get if they cut the rectangular prism in half using a diagonal cut along the top as shown by the purple line in the diagram to the right. Did you encounter anything that made it difficult to estimate theĮstablishing the formula for a triangular prism.Did you over-estimate or under-estimate the volume?.Were the estimates close to the actual volume?.These questions can be used for discussion once students have Have shaded each of the cross-sections they can use these to find theĪrea of the shaded portion and then multiply this by the length/height Introduce the formula for calculating volume as area of cross sectionĪsk students to use this formula to check their estimations. Isometric drawing paper to estimate the volume of their prisms asĪccurately as possible. You get when you cut through an object) and that the cross-sectionĪlong any part of the length needs to identical for a prism.Īsk students to shade one cross-section on each of their ‘prisms’ toĮnsure they can identify the cross section of each shape.Īs a final task, ask students to use the centimetre squares on their Walk around the classroom and check that students areĭrawing prisms with identical ends and straight sides.įollowing this activity revise what cross-section means (the shape (shapes that do not have a regular shaped cross-section) and to tryĭrawing one. Encourage students to think about irregular prisms Most likely students will draw regular prisms Rectangular prism, triangular prism, hexagonal prism etc. You may like to provide a list of prisms for students to draw, e.g. To draw different prisms using the definition stated. Provide each student with 1 cm isometric drawing paper. What terminology they may not understand in that definition and provide Identical ends, straight sides and the same cross-section. Introduce prisms to students as 3-dimensional shapes that have Them to identify and find the area of the cross section rather Depending on the rotation of the shape the front face The area of the ‘front face’ and multiply this by the length or Of cross-section first they will be able to calculate theĪ common misconception may arise if students are told to find Two hexagonal prisms are the same volume but are Whether the student ‘see’ the length or the height.Į.g. On the rotation of the shape will determine The cross section is, find the area of it and then Introduced as area of the cross section × length The cross section will be the same shape as the identical ends of the prism. Show how the cross section must be identical along the length (or height) of the shape.Īll these shapes are triangular prisms as they have identical triangular ends, straightĮdges and a uniform cross section. Rotations of the shape, so students become familiar with what is required to call a shape a When introducing new prisms such as a triangular prism show a variety of drawings and This formula only applies to rectangular prisms. Showing a justification for the formula will support student understanding that Rectangular prism made from 1 cm cubes or drawing one on a computer program such as Clarify with students how this formula is established by showing a Students may be familiar with the formula length × width × height to find the volume ofĪ rectangular prism. Prisms are three-dimensional shapes with identical ends that can be cut into identicalĬross sections all along their length or height. To develop formulas to calculate the volume of other prisms such as triangular prisms. The volume of a rectangular prism from ( VCMMG259). At this level, students will continue to develop their understanding of volume and finding


 0 kommentar(er)
0 kommentar(er)
